How many climbing centers should there be in a city?
Summary
My friend was wondering about if there were enough climbing centers per city depending on how many people lived in the city and here is my rudimentary attempt to try to answer that question without anything fancy.
Introduction
This project was started as a joint project between a friend that enjoys climbing and myself. He pointed out that he was convinced that the number of climbing centers in our city corresponded with it’s size. In fact, to climb he would usually travel to a larger city nearby.
Since I’ve been doing nothing but studying for my MCAT the past few months, I suggested that we see if could extract some relation between the number of people in a city and the number of climbing centers. I figured that this would be a good excuse to get back into math as I haven’t done any stats projects since university.
My gut feeling was that we should see greater ‘crowding’ or density of climbing centers serving people in the more crowded cities than in the smaller ones. And I made this guess based on city size and the assumption that climbing center availability is based solely on the city size and not city politics or inhabitant influence or the myriad of other factors that could affect the presence of a climbing center in a city or not.
To be honest, I haven’t the slightest idea what is a normal number of people for a single climbing center in an area neither for the number of climbing centers for people.
Methods and Calculations
My friend found the initial table and that he found the table on Wikipedia and as soon as I can find the link or he sends it to me, I will cite the initial data .
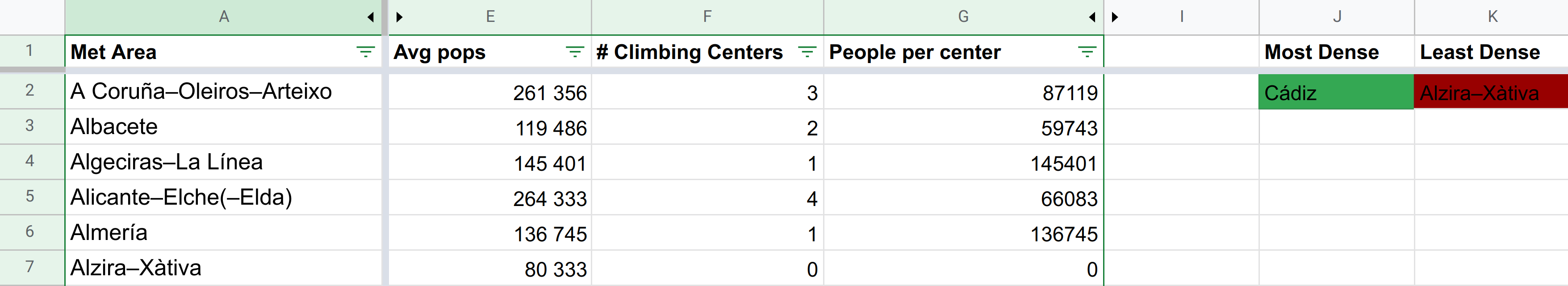
The table features a variety of headers including city Met. Area, pop(ESPON), Urban Audit, Ruiz, and Avg. pop. For the calculations only the average population was used and for determining the number of climbing centers, the Met. Area was used. Some of these labels feature more than one city center.To make things more confusing, it isn’t clear on the table how the Avg. pop was calculated
Each city was manually located on google maps and using its search function, the number of climbing centers was counted within the ‘smart radius’ that google maps offers when one searches a city for a place. The number of climbing centers was then counted and recorded on the spreadsheet. For a Met. Area with more than one city center, the smallest suggested area by google maps was used to count the number of climbing centers e.g. A Coruña–Oleiros–Arteixo together had 3 climbing centers.
I then divided the average population was then divided by the number of climbing centers to obtain a density.
For the cities with no climbing centers a density of 0 was assigned to them so that there was no division by zero.
Results
I found that of all Met. Area(s), Cadiz unexpectedly was the most dense of the cities with 275,154 people per climbing center (ppcc) while the least dense city with at least one climbing center was Torrelavega with 27,279 ppcc. Met. Area(s) with no climbing centers were excluded as density wouldn’t make any sense.
Excel header taken from spreadsheet
Problems Faced
Density score interpretation
- The density score is a ratio of the average population to the number of climbing centers in a given Met area and so it represents a type of crowding or corralling of potential climbers into a climbing center.
0’s in the data set
- There are Met. Area(s) with no climbing centers and so as mentioned before, they were assigned a density of zero. This isn’t really realistic though as if someone wants to go to climbing center, in some cases they would be able to arrive using private or public transportation. As evidence, despite having a climbing center here in our city, my friend commutes to the next larger one to climb.
Climbing Center Counting
- It’s possible that some climbing centers were designed to function between multiple cities/pueblos and such design was not taken into account during counting. Furthermore, as some cities are so close together, some climbing centers may have been counted more than once while other, smaller/newer climbing centers were not counted at all.
Others
- As google maps city view was used to determine the circumference around each city where climbing centers were searched, the radii are inconsistent. This leads to another problem as the people counted for each geographic area should be within the circumference.
Next Steps
In future projects I would like to control for the for the area measured by restricting the population and the climbing centers to the same geographic region. The latter half of this can be achieved by using google’s map API while the former may be done through using census data.
The density score is strange when you look at it from the actual level of a city or town. A city can be divided in such a way that perhaps private transport is reasonable and the public transport to get to a climbing center is complicated in which case it is essentially inaccessible.To better understand what this number means it would need to be compared to the average number of people assigned to a single building. Such question should be made to perhaps an architect or civil engineer.
Within Spain, in future explorations it would be a good idea to compare between cities based on their GDP or average income or other demographic factors that may be behaving as confounding or mediating variables.